How many halves fit into a quarter? Half of them
✅ Math is hard
❌ This math is hard
p/q=q
So q=√p
Works with a lot of numbers ☝🏻🤓
0.25 / 0.5 = 0.5
0.25 = 0.5 × 0.5
1/4 = 1/2 × 1/2If you give half a person a quarter of a thing, how much would you be giving a full person? That’s right baby, half a thing. Don’t sweat it.
x / sqrt(x) = sqrt(x)
Damn who would’ve thought?
I worry that people who can get onto the internet find fractions a challenge.
I don’t think this was shared because people are finding it a “challenge” it just looks funny.
It takes all of a few seconds for your actual mathematical processing to kick in and you go “oh yeah duh” but its just a funky little string of numbers.
It lives in the same camp as how none of the >3 whole multiples of 17 feel like multiples of 17. 68? Preposterous.
It’s why my favorite way to troll the usual “why isn’t everyone on metric” goombahs is to tell them they’re just too lazy and/or dumb to do math with fractions.
I mean, I’m too lazy, but really - why make life harder than it has to be?
It isn’t actually harder. At all. People just think it is because them funny / signs is different from regular math. So they get put off by it even if they’re actually good at it because they’ve built the idea of hating fractions even though it’s a very intuitive thing.
You take a string, fold it in half, you’ve got a fraction in front of you. The rest follows from that basic principle. But when you put it on paper, the only thing that isn’t obvious is dividing fractions. Even then, you could figure it out on your own with a bit of thought.
Unfortunately, you jam a bunch of kids in a room and make them do boring things, often being taught by someone that isn’t actually good at math, and may have no desire to teach math in the first place, and you get droves of kids that hate math. Someone that likes math, and has spent time playing with it, they’ll have a way of translating it into different terms. Instead, you go by the book regardless of if the book works for kids of a given age.
Fractions are just as easy as decimal. You can’t imagine how many kids struggle with division in decimals, or even just keeping the number line in mind when dealing with them.
The one belt benefit decimal has over fractions is the ability to write things out by line and do most problems (other than division) in a simple box. That goes away once you’re dividing though. Dividing fractions is easier for some.
Also, fractions are easier to estimate with. You can almost always guesstimate what half of a thing will be, so you can almost always keep going until the fraction is too small visually to detect. Eyeballing a tenth of something is not as easy for most people.
Besides, it’s good for your brain. It’s like a muscle in that regard. If you don’t use it, it gets flabby. Flabby brains lead to shitty thinking.
I’m lazy, that’s why I prefer metric. It’s infinitely easier than dealing with fractions.
I just think of division as how many times the right expression fits inside the left expression. 0.5 fits into 0.25 only 0.5 aka 1/2 times, because only half of it fits.
Precisely this. The people not getting the OP are why Common Core was developed.
The numbers between zero and one are where all of the fun is!
1×2=2
Wow. Much brain. Maths wow.
25/5=5
2.5/0.5=5
o.O
I think, it is the real world logic that makes it hard to grasp. If you divide something with something small it becomes bigger. Mathematically it’s easy and makes sense, but it it’s somehow not intuitive. Especially for young me :)
That’s the same as 2/2=1 3/3=1 268/268=1 …
Except that’s not what’s written.
It’s 0.25/0.5
Raising it exponentially would be
2.5/5, or
25/50=50
1/2≠50. Does not compute.
A quarter is one half of one half. Makes perfect sense.
This just comes down to the fact that “dividing by a fraction is the same as multiplying by the inverse of the fraction” is an easy rule to follow but not particularly intuitive. In natural language, when most people hear “divide by half” they’re actually picturing “divide by two” in their head.
This don’t avoid to sleep not even for 1/2 second. But pick any number. If that number is even, divide it by 2. If it’s odd, multiply it by 3 and add 1. Now repeat the process with your new number. If you keep going, you’ll eventually end up at 1.
You can’t prove that!
Yes, but everyone tried since a century to find a number with which it don’t work, good to avoid sleep.
It’s going to be okay:
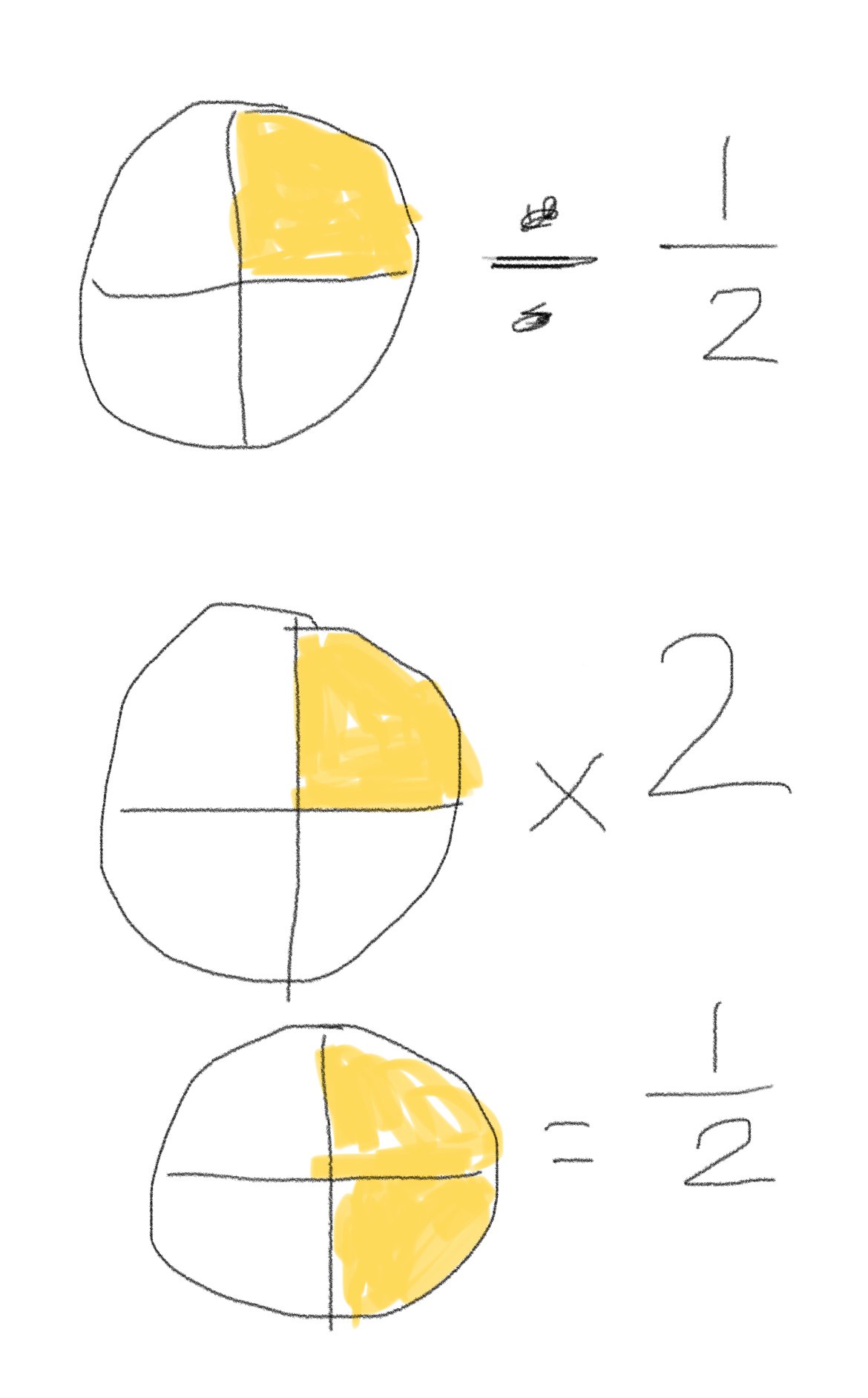
Edited to add this: Singapore math insists however, that we eliminate the use of visuals in describing arithmetic within the rationals. They encourage that users of common core rely upon the number line, and solely the number line for thorough and most mathematically sound representations of arithmetic, even when involving the division of fractions.
For those not up to speed to with common core, remember how the teacher used to draw a diagram of a bunny hopping from one integer to the next integer to represent adding given integers, such as 4+3, or -2+1? Imagine that representation being used with problems like 1/7 divided by 5/49, and no decimal approximation is allowed. It’s fascinating and truly something to appreciate from the standpoint of someone who truly loves mathematics. I think it makes for great discussions amongst math graduates like myself, and other math enthusiasts. What does that mean for those who are not so enthused? Sometimes it means the teacher receives death threats from angry students. You can’t make everyone happy.
I’m not sure I completely agree with the number-line-only approach, but I’m definitely sympathetic to it. It reinforces the idea that fractions are numbers like any other numbers, and not pieces of pizza.
I get that. I like the number line approach, and respect it, but I have also observed seasoned math coaches fumble the visual explanation of a division by fractions problem where the numerators and denominations were relatively prime. As soon as the guy had drawn the first fraction and began to say, “we’d multiply by the recipro-…”, I could tell it was going to be long problem. He just stood there, and then asked, “well, how would I go about explaining the ‘keep change flip’, if you will?” He ended the problem by saying he might just explain that the distance drawn for the first fraction needs to be repeated on the other side of the fraction to show the multiplication by the denominator of the second fraction, and then that distance could be broken into parts to demonstrate the division by the previous numerator of the second fraction.
Basically he ended the problem by saying, “let’s just reflect it! Then we can break it up.” There wasn’t really a sound justification for the reflection piece of the process, other than saying, “we need to multiply by the reciprocal of the second fraction, so we’ll just have to multiply by its denominator it had, prior to flipping it.”
That was the quietest meeting I have ever seen amongst that group of adults.











